
The points in the original figure and the flipped or mirror figure are at equal distances from the line of reflection.ġ). For example, consider a triangle with the vertices $A = (5,6)$, $B = (3,2)$ and $C = (8,5)$ and if we reflect it over the x-axis then the vertices for the mirror image of the triangle will be $A^) = (-5, 1)$ When we reflect a figure or polygon over the x-axis, then the x-coordinates of all the vertices of the polygon will remain the same while the sign of the y-coordinate will change. The reflection of any given polygon can be of three types: This is a different form of the transformation. steps Final answer Step 1/3 To reflect a point or a vertex over the x a s. Apply a reflection over the line x-3 Since the line of reflection is no longer the x-axis or the y-axis, we cannot simply negate the x- or y-values. We can perform the reflection of a given figure over any axis. The formula for reflection over the x-axis is to change the sign of the. Simple reflection is different from glide reflection as it only deals with reflection and doesn’t deal with the transformation of the figure. We can draw the line of reflection according to the type of reflection to be performed on a given figure. The process of reflection and the line of reflection are co-related. So if we have a graphical figure or any geometrical figure and we reflect the given figure, then we will create a mirror image of the said figure. Read more Prime Polynomial: Detailed Explanation and ExamplesĪ reflection is a type of transformation in which we flip a figure around an axis in such a way that we create its mirror image. The most important feature during this reflection process is that the points of the original figure will be equidistant to the points of the reflected figure or the mirror figure/image.Īs the points of the original polygon are equidistant from the flipped polygon, if we calculate the mid-point between two points and draw a straight line in such a manner that it is parallel to both figures, then it will be our line of reflection. Only the direction of the figures will be opposite. The same is the case with geometrical figures.įor example, if we have a polygon and we reflect it along an axis, then you will notice that the shape and size of both figures remain the same. Algebra a) create a graph of the function y10x over -2 < x < 2 (provide a.

For example, if you raise your right arm, then you will observe that your image will also be raising his right arm, but that the right arm of the image will be in front of your left arm. Explanation: Our goal is too change this equation y 1 3(x +6) into a.
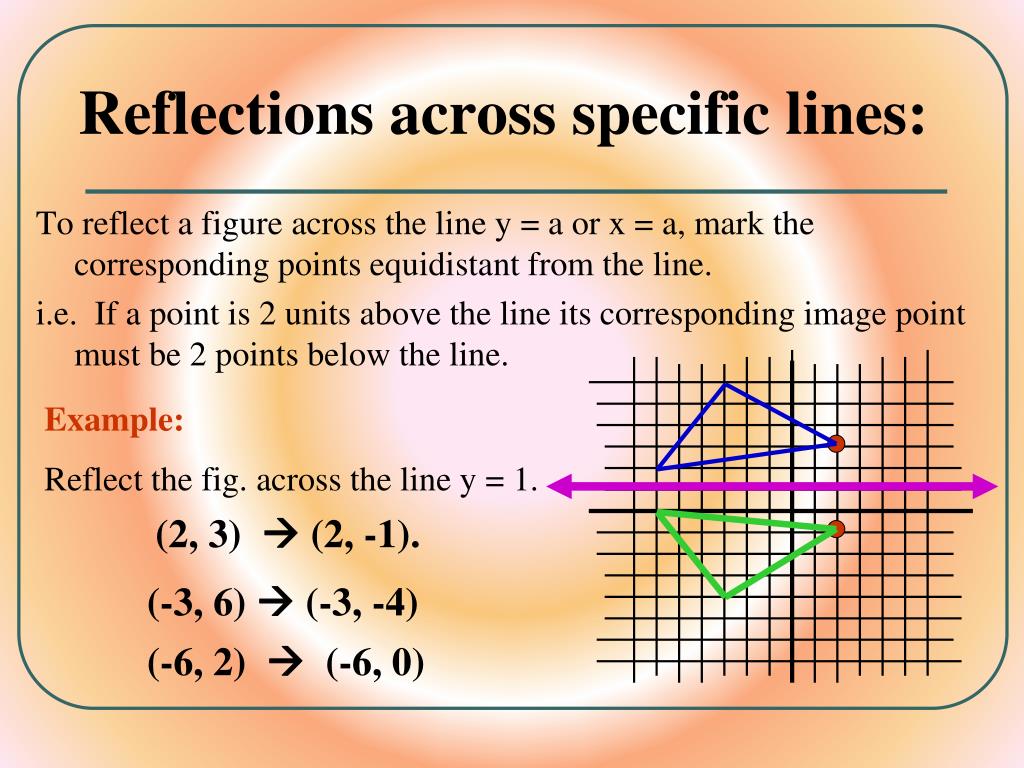
Say you are standing in front of a mirror the image of yourself in the mirror is a mirror image. Let’s first discuss what is meant by a mirror image.
#Reflection across x 3 formula plus#
Read more y = x^2: A Detailed Explanation Plus Examples


 0 kommentar(er)
0 kommentar(er)
